Fusing the best, from the best

A new IOL formula is dynamic, quick and accurate.

By Aazim A. Siddiqui, MD and John G. Ladas, MD, PhD

“It’s masochism for a mathematician to do without pictures… [Otherwise] how can they see the relationship between that motion and this.” — James Gleick, Author, “Chaos”

For every cataract procedure you perform, there exists at least one commonality: You choose and review IOL calculations. It could be three charts or it could be 30, but you pick a formula for a given eye. Perhaps you already have a certain mental “algorithm,” or you use one formula for every patient — for most of us, the latter is typically the case.

The printout from your biometer typically spews multiple pages of input data and formulas. How many, and which formulas, will you look at? Was the K reading flat or steep? Is the axial length long or short? Does the anterior chamber depth (ACD) matter? Is a Koch adjustment called for? The problem: the three third-generation formulas you have selected each produce a different IOL power. Should you check the more complex fourth-generation formulas to compare? If the results are different, then which one do you choose?

As you prepare to perform a delicate surgery, you do not want any confusion regarding IOL selection for this particular eye — which differs from every other you will see that day.

You also don’t want to spend extraordinary amounts of time on making the IOL decision; this should not take longer than the surgery, especially if you aren’t sure about the verdict. Regardless of how many times you repeat this process during the week, be it three or 30 times, spending more time than necessary amounts to lost time, and that is a valuable commodity.

Thankfully, this may have all come to an end.

An amalgamated formula

The IOL calculation methods surgeons use have steadily improved over the past few decades. It wasn’t until the emergence of theoretical formulas such as Holladay I, SRK/T and Hoffer Q that IOL calculation significantly improved.

In this era, the expectation is these types of formula will allow the majority of eyes to within 0.5 diopter of the target refraction. That said, these sophisticated formulas are known to do well under certain circumstances but have displayed weaknesses in others. By representing, however, these formulas in three dimensions, we take the best parts of all these formulas and leave alone their weaknesses. In other words, our formula, across all types of eyes, maximizes the use of existing formulas.

This methodology, published in the October issue of JAMA Ophthalmology shows that we can generate a single 3D “super surface” by using a 3D representation of IOL formulas, thus creating an ideal IOL “super formula.”¹

Real-life examples

Let’s explore how this works with a typical real life example.

The super formula interface localizes to the correct region and provides an accurate IOL power value (Figure 1). It automatically takes into account the axial length, keratometry, A-constant, target refraction and whether a measured ACD value will be used. The correct portion of the surface is identified to determine the most appropriate IOL power.

Figure 1. The super formula interface localizes to the correct region and provides an accurate IOL power value.

Other scenarios highlight the versatility of the super formula and its time-saving capabilities.

For example, a small eye with short axial length is notoriously difficult to calculate with a high degree of accuracy because a very small shift in the effective IOL lens position can change the calculations dramatically. In published literature, the Hoffer Q or a fourth-generation formula such as the Haigis are known to perform reasonably well in these eyes.

The Ladas super formula would localize variables onto the Ladas super surface and provide an accurate calculation and appropriate selection of the lens.

In a small eye with a short axial length, the Ladas Super Formula Calculator determines the best IOL power based on the power of many previously published studies (Figure 2).² Note that the section of the Ladas super surface graph for short axial lengths is very steep, which indicates that slight changes in the axial length can have large effects on IOL power.

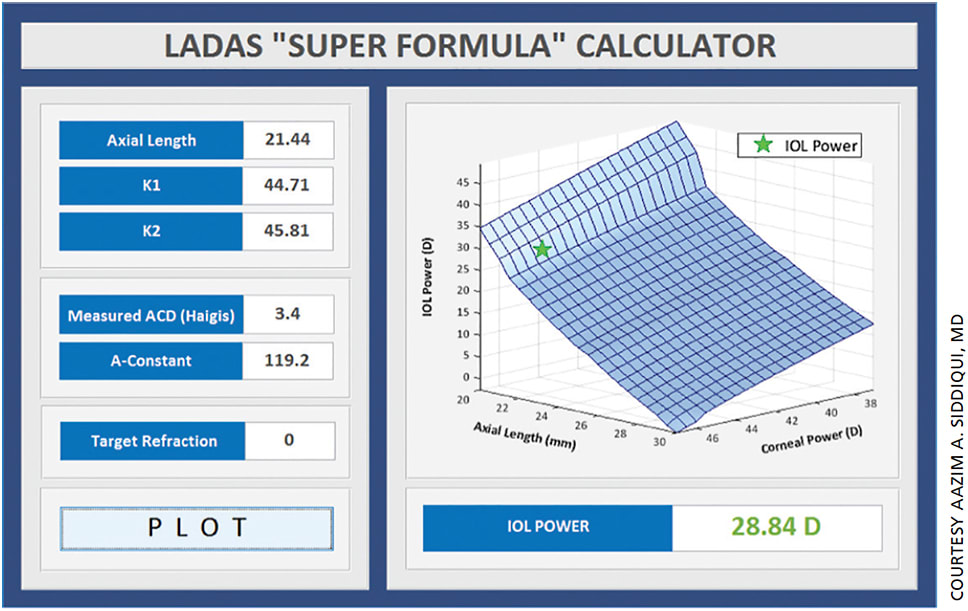
Figure 2. In a small eye with a short axial length, the Ladas Super Formula Calculator determines the best IOL power based on the power of many previously published studies.² Note that the section of the Ladas super surface graph for short axial lengths is very steep, which indicates that slight changes in the axial length can have large effects on IOL power.

Also, in myopic eyes with axial lengths greater than 25.0 mm, our calculation results have tended to be less accurate unless we remember to apply the Wang-Koch adjustment to the axial length before doing the calculation. With the Ladas super formula, that axial length is calculated automatically.³ (When the authors note further refinements, those will be updated into the Ladas super formula.)

In Figure 3, a highly myopic eye utilized the Wang-Koch axial length adjustment; Wang-Koch is already incorporated into the Ladas super formula. (calculated soon at www.IOLcalc.com)

Figure 3. This highly myopic eye utilized the Wang-Koch axial length adjustment; Wang-Koch is already incorporated into the Ladas super formula (calculated soon at www.iolcalc.com).

The algorithm covers negative power IOLs, short eyes, long eyes, average eyes and high Ks.

Other real-life examples

To demonstrate this concept’s utility, we will present a couple of cases performed by Uday Devgan, MD, a co-author of the JAMA paper. The IOL power was calculated using the super formula.

Here’s a patient with a commonly seen eye:

Axial length = 23.80 mm; K1 = 44.23; K2 = 45.73; ACD = 2.29 mm

Based on these parameters, the super formula automatically will localize to a particular part of the super surface, which has picked the most appropriate IOL based on the axial length. The other formulas differed in their results, giving options of 18.0, 18.5, or 19.0 D for a plano outcome. The super formula’s calculation? 17.95 D.

Next, let’s consider a more complex scenario:

Axial length = 32.55 mm; K1 = 44.47; K2 = 45.30; ACD = 3.70 mm

Based on these parameters, the super formula recognized the highly myopic nature of the eye with an exceedingly long axial length. It automatically localized the solution to a particular part of the super surface using the Haigis formula because it was a negative IOL. No other formulas were within at least 0.5 diopters. Yes, the Koch adjustment, which also could have been used at the extremes of the axial length, but was more than 1 diopter different than the Haigis, thus creating a clinical dilemma.

These clinical disparities are significant enough to potentially cause a change in clinical outcomes; this potential further confirms the value of using the super formula. By using the IOL power value from the super formula, Dr. Devgan could pick the most appropriate lens for his patient, which was -1.32 D. “Both [results] turned out to be perfect,” says Dr. Devgan.

He adds: “We are looking to further hone the accuracy of results based on the experiences of users. For example, you could do an IOL calc on our website* and then report back with the actual results to tell us how we did. We use that data [from thousands of eyes] to further hone the methods to make it even more accurate.”

(At the AAO annual conference last month, Dr. Ladas told OM that the group started by incorporating four formulas, and now will apply an optimum strategy to the whole surface. At least 10 major cataract and refractive surgical groups will supply outcome data to allow his team to embed other variables, like anterior chamber depth, into the formula.)

Practice management benefits

We also feel that the super formula is an incredible time-saver. Every surgeon will know how much time he spends deciding on which formulas to use for each patient. A surgeon with 10 slated cataract surgeries, who spends 5 to 10 minutes on calculations per patient, would save more than an hour if she uses the super formula.

While we believe this will help you in your practice, we also believe that this is an important teaching tool for future surgeons. The concept of choosing a particular formula in a given situation is foreign to most residents. It is impossible to have them commit an algorithm to memory.

Dr. Devgan is using the calculator with his residents. Its simplicity allows him to review the IOL calculations graphically with each resident. He can discuss why a particular formula is chosen for that particular patient and the refractive target. As the students begin to fully grasp the model of making IOL calculation, “the concept of a 3D Super Surface and a super formula would simplify their surgical decision-making as well as improving patient refractive outcomes,” he says.

Conclusion

While the super formula provides a simple solution to a current problem, could it get even better? By using a three-dimensional graphical surface, we can optimize all of its regions in a discrete manner. The main advantage of the super surface and super formula is the fact that it can evolve over time. Every “inch” of the super surface, and consequently the super formula, can be refined based on empirical data.

The idea is not only to make our current methods better, but also to create a system in which the super formula never ceases to improve.

With this formula, we hope to relieve every cataract surgeon’s headache that always involves choosing the most appropriate IOL formula for any given eye. By imagining the modern IOL formulas as three-dimensional entities, a surgeon can take the best parts of each formula and create one optimal super surface and formula. With this singular super formula, you can calculate the most appropriate IOL power value for eyes with any level of diversity in axial length, K and ACD. We hope this formula via continued improvements will provide superior patient outcomes. OM

*For more information, visit www.iolcalc.com.

REFERENCES

About the Authors
	Aazim Siddiqui is a medical school graduate, researcher, and an aspiring ophthalmologist with a special interest in technology and mathematics. He is currently involved with ophthalmic research focused around intraocular lens formulas and calculations, where the aim is to improve the process of IOL calculations and optimize accuracy and lead to improved patient outcomes. He is the co-inventor of and mathematician behind the IOL “Super Surface” and “Super Formula.”
	John Ladas, MD, PhD, is surgeon/director at Maryland Eye Consultants and Surgeons. He is also an assistant Professor of Ophthalmology for Research, Wilmer Eye Institute, Baltimore, MD. He can be reached at jladas@marylandeye.com.